 Calculus of Variations Summer Term 2014
Calculus of Variations Summer Term 2014
Purpose of Lesson: To consider several problems with inequality constraints c. Daria Apushkinskaya. 2014 (). Calculus of variations lecture 9. 23. Mai 2014. 2 /
 Introduction to the calculus of variations
Introduction to the calculus of variations
The calculus of variations is a hugely important topic in the natural sciences. It leads naturally to the Lagrangian formulation of mechanics mentioned above
 Calculus of Variations Summer Term 2014
Calculus of Variations Summer Term 2014
̂J[·] is a function of the vector y = (y1y2
 Calculus of Variations Summer Term 2014
Calculus of Variations Summer Term 2014
The last equation just gives you back your constraint. c. © Daria Apushkinskaya. 2014 (). Calculus of variations lecture 6. 23
 Lecture 19. Direct Methods of the Calculus of Variations.
Lecture 19. Direct Methods of the Calculus of Variations.
The classical theory of Calculus of Variations roughly covers the time from Euler to the end of 19th century is concerned with so-called Indirect Methods. The
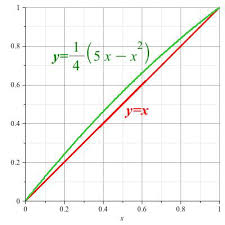 Calculus of Variations Summer Term 2014
Calculus of Variations Summer Term 2014
We need an analogous process for extremal curves as well. c. Daria Apushkinskaya. 2014 (). Calculus of variations lecture 8. 23.
 Calculus of Variations Summer Term 2014
Calculus of Variations Summer Term 2014
But can we apply the Euler-Lagrange equations? c. Daria Apushkinskaya. 2014 (). Calculus of variations lecture 11. 6.
 7.2 Calculus of Variations
7.2 Calculus of Variations
This section is also the opening to control theory—the modern form of the calculus of variations. Its constraints are differential equations and Pontryagin's.
 Materials Science-inspired problems in the Calculus of Variations
Materials Science-inspired problems in the Calculus of Variations
Thilo Simon. ”Rigidity of branching microstructures in shape memory alloys”. ArXiv e-prints (2017) arXiv: 1705.03664. • Thilo Simon.
 The Calculus of Variations
The Calculus of Variations
21.03.2021 2. Examples of Variational Problems. The best way to appreciate the calculus of variations is by introducing a few concrete examples of both ...
 Brief notes on the calculus of variations
Brief notes on the calculus of variations
The fundamental lemma of the calculus of variations. 4. 5. The Euler–Lagrange equation. 6. 6. Hamilton's principle of least action.
 The Calculus of Variations
The Calculus of Variations
21-Mar-2021 The Calculus of Variations. Peter J. Olver. School of Mathematics. University of Minnesota. Minneapolis MN 55455 olver@umn.edu.
 7.2 Calculus of Variations
7.2 Calculus of Variations
This section is also the opening to control theory—the modern form of the calculus of variations. Its constraints are differential equations and Pontryagin's.
 Calculus of Variations
Calculus of Variations
In calculus of variations the basic problem is to find a function y for which the functional I(y) is maximum or minimum. We call such functions as extremizing
 calculus of variations: minimal surface of revolution
calculus of variations: minimal surface of revolution
SiqiClover.pdf
 Calculus of Variations
Calculus of Variations
Calculus of Variations. Lecture Notes. Erich Miersemann. Department of Mathematics. Leipzig University. Version October 2012
 Karen Uhlenbeck and the Calculus of Variations
Karen Uhlenbeck and the Calculus of Variations
and the Calculus of Variations. Simon Donaldson. In this article we discuss the work of Karen Uhlenbeck mainly from the 1980s
 BASICS OF CALCULUS OF VARIATIONS 1. Brachistochrone
BASICS OF CALCULUS OF VARIATIONS 1. Brachistochrone
BASICS OF CALCULUS OF VARIATIONS. MARKUS GRASMAIR. 1. Brachistochrone problem. The classical problem in calculus of variation is the so called
 Introduction to the calculus of variations
Introduction to the calculus of variations
mathematical apparatus called the calculus of variations: this is the main purpose of this unit. In ordinary calculus we often work with real functions
 Appendix A - Functionals and Calculus of Variations
Appendix A - Functionals and Calculus of Variations
1 Following the same analogy within the realm of the functionals calculus of variations (or variational calculus) is the counterpart of differential calculus
 [PDF] Calculus of Variations
[PDF] Calculus of Variations
Integration by parts in the formula for g (0) and the following basic lemma in the calculus of variations imply Euler's equation
 [PDF] calculus of variations
[PDF] calculus of variations
The present course is based on lectures given by I M Gelfand in the Mechanics and Mathematics Department of Moscow State University
 [PDF] Calculus of Variations - IIST
[PDF] Calculus of Variations - IIST
In Calculus of Variations we will study maximum and minimum of a certain class of functions Equation (2) is known as the Euler-Lagrange equation
 [PDF] Introduction to the calculus of variations - The Open University
[PDF] Introduction to the calculus of variations - The Open University
This format ensures that mathematical notation is presented accurately and clearly The PDF of this extract thus shows the content exactly as it would be seen
 [PDF] The Calculus of Variations - www-userscsumnedu
[PDF] The Calculus of Variations - www-userscsumnedu
21 mar 2021 · In these notes we will develop the basic mathematical analysis of nonlinear minimization principles on infinite-dimensional function spaces — a
 [PDF] 72 Calculus of Variations - MIT Mathematics
[PDF] 72 Calculus of Variations - MIT Mathematics
The “Euler-Lagrange equation” ?P/?u = 0 has a weak form and a strong form carries ordinary calculus into the calculus of variations
 [PDF] Calculus of variations and its applications
[PDF] Calculus of variations and its applications
In this thesis the calculus of variations is studied We look at how opti- mization problems are solved using the Euler-Lagrange equation Functions
 [PDF] Bruce van Brunt - The Calculus of Variations
[PDF] Bruce van Brunt - The Calculus of Variations
The calculus of variations has a long history of interaction with other branches of mathematics such as geometry and differential equations
 [PDF] Calculus of Variations - Physics Courses
[PDF] Calculus of Variations - Physics Courses
1 oct 2009 · Calculus of Variations 1 Functional Derivatives The fundamental equation of the calculus of variations is the Euler-Lagrange equation
![[PDF] Calculus of Variations - Physics Courses [PDF] Calculus of Variations - Physics Courses](https://pdfprof.com/Listes/17/24065-17Calc_Var.pdf.pdf.jpg)
Calculus of Variations
Joel G. Broida
University of Colorado, Boulder
Copyright
c?2009 by Joel G. Broida. All rights reserved.October 1, 2009
0Calculus of Variations
1 Functional Derivatives
The fundamental equation of the calculus of variations is the Euler-Lagrange equation d dt? ∂f∂x? -∂f∂x= 0. There are several ways to derive this result, and we will cover three of the most common approaches. Our first method I think gives the most intuitive treatment, and this will then serve as the model for the othermethods that follow. To begin with, recall that a (real-valued)functiononRnis a mapping f:U?Rn→R. In other words,ftakes apointin some subsetUofRn and gives back a number, i.e., a point inR. In particular, the domain offis a subset ofRn. We write this mapping asf(x). In contrast to this, afunctionalFis a "function" whose domain is the space ofcurvesinRn, and hence it depends on theentire curve, not just a single point. Very loosely speaking, we will take acurveto be a differentiable mappingy:U?Rn→Rm. So a curve is just a function defined on some interval, and a functional is a "function of a function." For example, lety(x) be a real valued curve defined on the interval [x1,x2]?R. Then we can define a functionalF[y] by
F[y] :=?
x2 x1[y(x)]2dx?R.
(The notationF[y] is the standard way to denote a functional.) So a functional is a mapping from the space of curves into the real numbers. We now want to define the derivative of such a functional. There are several ways to go about this, and we will take the most intuitive approach that is by analogy with the usual notion of derivative. So, letf(t) be a function of a single real variable, and recall the definition of the derivativef?(t): f ?(t) =df dt(t) = limh→0f(t+h)-f(t)h.(1) This is equivalent to saying thatfis differentiable attif there exists some numberL(called thederivativeoffatt) and a function?with the property that lim h→0?(h) h= 0 such that f(t+h) =f(t) +Lh+?(h).(2) 1 Before proving the equivalence of these formulations, let me make two re- marks. First, we say that such a function?(h) isO(h2) (orderh2). And second, note that the numberLis just a linear map fromRtoR. (In this case, L:R→Ris defined byL(h) =Lhforh?R.) In fact, it is this formulation of the derivative that is used to generalize differentiation tofunctions fromRnto R m, in which case the linear mapLbecomes the Jacobian matrix (∂yi/∂xj). Let us now show that equations (1) and (2) are equivalent. Note that if we start from (1) anddefinethe function?by ?(h) =? f(t+h)-f(t)-f?(t)hforh?= 00 forh= 0
then f(t+h) =f(t) +Lh+?(h) whereL=f?(t) and (by equation (1)) lim?(h)/h= 0. Conversely, if we start from equation (2), then f(t+h)-f(t) h=L+?(h)h and taking the limit ash→0 we see thatf?(t) =L. Now let us return to functionals. Letγbe a curve in the plane:γ={(t,x) :x(t) =xfort0< t < t1}.
Let?γbe an approximation toγ, i.e.,
?γ={(t,x) :x=x(t) +h(t)} for some functionh(t). We abbreviate this by?γ=γ+h. LetFbe a functional and consider the differenceF[?γ]-F[γ] =F[γ+h]-F[γ]. t0t1x tγeγ
We say thatFisdifferentiableif there exists a linear mapL(i.e., for fixedγ we haveL(h1+h2) =L(h1)+L(h2) andL(ch) =cL(h)) and a remainderR(h,γ) with the property thatR(h,γ) =O(h2) (i.e., for|h|< εand|h?|=|dh/dt|< ε we have|R|F[γ] =?
t1 t0f(x(t),x(t),t)dt
is differentiable and its derivative is given byL(h) =?
t1 t 0? ∂f ∂x-ddx? ∂f∂x?? hdt+∂f∂xh????t 1 t 0(4) Proof.Sincefis a differentiable function we have (using equation (2) in the case wherefis a function of the two variablesxand x)quotesdbs_dbs2.pdfusesText_3[PDF] sous espace propre
[PDF] calcul vectoriel dans le plan tronc commun
[PDF] symbole produit vectoriel word
[PDF] exercice produit vectoriel corrigé
[PDF] produit vectoriel science de l'ingénieur
[PDF] dimensionnement d'un vérin hydraulique double effet
[PDF] calcul verin hydraulique
[PDF] dimensionnement vérin pneumatique
[PDF] calcul verin hydraulique xls
[PDF] logiciel calcul verin pneumatique
[PDF] dimensionnement d'un verin hydraulique pdf
[PDF] tableau force verin hydraulique
[PDF] calcul force vérin pneumatique
[PDF] exercice corrigé vérin hydraulique
