Week #3 - Exponential Functions and Logarithms; The Derivative

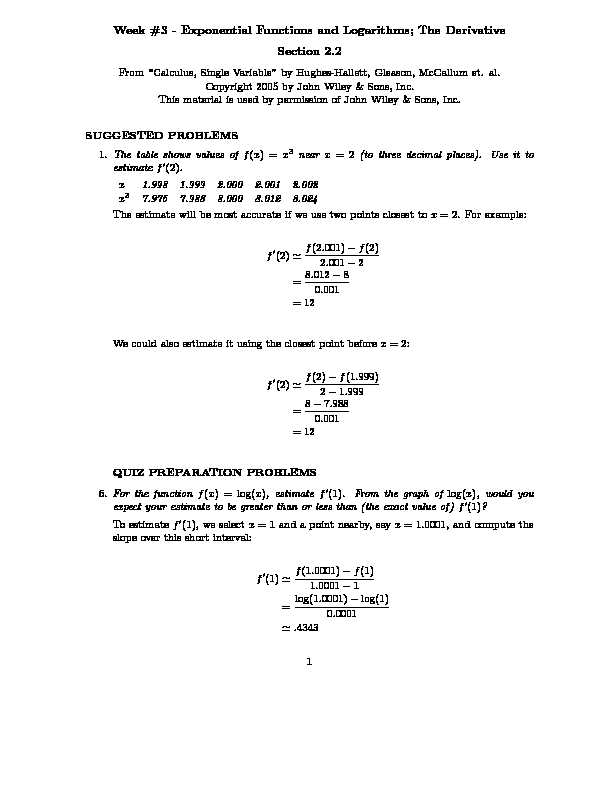213273 Week #3 - Exponential Functions and Logarithms; The Derivative

Section 2.2

From "Calculus, Single Variable" by Hughes-Hallett, Gleason, McCallum et. al.

Copyright 2005 by John Wiley & Sons, Inc.

This material is used by permission of John Wiley & Sons, Inc.

SUGGESTED PROBLEMS

1.The table shows values off(x) =x3nearx= 2(to three decimal places). Use it to

estimatef?(2). x1.998 1.999 2.000 2.001 2.002 x

37.976 7.988 8.000 8.012 8.024

The estimate will be most accurate if we use two points closest tox= 2. For example: f ?(2)?f(2.001)-f(2)

2.001-2

8.012-8

0.001 = 12 We could also estimate it using the closest point beforex= 2: f ?(2)?f(2)-f(1.999)

2-1.999

8-7.988

0.001 = 12

QUIZ PREPARATION PROBLEMS

6.For the functionf(x) = log(x), estimatef?(1). From the graph oflog(x), would you

expect your estimate to be greater than or less than (the exact value of)f?(1)? To estimatef?(1), we selectx= 1 and a point nearby, sayx= 1.0001, and compute the slope over this short interval: f ?(1)?f(1.0001)-f(1)

1.0001-1

log(1.0001)-log(1)

0.0001

?.4343 1

0.8 1.0 1.2 1.4 1.6

-0.10 0.00 0.10 0.20 x f(x) = log(x) The graph of log(x) is concave down, as shown in the graph above. This means thatany instantaneous slope estimated by an interval slope will be anunderestimate(less steep) compared to the instantaneous slope. Sof?(1)>0.4343.

7.Estimatef?(2)forf(x) = 3x. Explain your reasoning.

To estimatef?(2), we selectx= 2 and a point nearby, sayx= 2.0001, and compute the slope over this short interval: f ?(2)?f(2.0001)-f(2)

2.0001-2

3(2.0001)-32

0.0001

?9.888

14.Show how to represent the following on Figure 2.23.

(a)f(4) (b)f(4)-f(2) (c) f(5)-f(2) 5-2 (d)f?(3)

Figure 2.23

2 (a) Height of the function atx= 4. (b) Difference in height betweenf(4) andf(2) (c) Slope/average rate between the points atx= 2 andx= 5. (d) Slope of the tangent line atx= 3

15.For each of the following pairs of numbers, use Figure 2.23 todecide which is larger.

Explain your answer.

(a) or? (b) or? 3 (c)or? (d) or? (a) These both representyvalues, andf(4) is clearly higher thanf(3). (b) These represent differences inyvalues betweenx= 2 and 3, compared tox= 1 and

2. Since the graph is getting flatter further to the right, that means the graph is

growing less quickly, or the samexdistance results in a smallerychange. Thus, f(2)-f(1)> f(3)-f(2) (c) These represent the slopes betweenx= 1 and 2, compared to the slope between x= 1 and 3. By skethching those lines on the graph, the fact thatthe curve is flattening to the right means that the slope over the longer interval will be lower than the slope over the shorter interval. Thus, f(2)-f(1)

2-1>f(3)-f(1)3-1

(d) These are the instantaneous rates of change / slopes of tangent lines atx= 1 and x= 4. Clearly, the graph has a slower rate of change further to the right, so f ?(1)> f?(4) 4 Week #3 - Exponential Functions and Logarithms; The Derivative

Section 2.2

From "Calculus, Single Variable" by Hughes-Hallett, Gleason, McCallum et. al.

Copyright 2005 by John Wiley & Sons, Inc.

This material is used by permission of John Wiley & Sons, Inc.

SUGGESTED PROBLEMS

1.The table shows values off(x) =x3nearx= 2(to three decimal places). Use it to

estimatef?(2). x1.998 1.999 2.000 2.001 2.002 x

37.976 7.988 8.000 8.012 8.024

The estimate will be most accurate if we use two points closest tox= 2. For example: f ?(2)?f(2.001)-f(2)

2.001-2

8.012-8

0.001 = 12 We could also estimate it using the closest point beforex= 2: f ?(2)?f(2)-f(1.999)

2-1.999

8-7.988

0.001 = 12

QUIZ PREPARATION PROBLEMS

6.For the functionf(x) = log(x), estimatef?(1). From the graph oflog(x), would you

expect your estimate to be greater than or less than (the exact value of)f?(1)? To estimatef?(1), we selectx= 1 and a point nearby, sayx= 1.0001, and compute the slope over this short interval: f ?(1)?f(1.0001)-f(1)

1.0001-1

log(1.0001)-log(1)

0.0001

?.4343 1

0.8 1.0 1.2 1.4 1.6

-0.10 0.00 0.10 0.20 x f(x) = log(x) The graph of log(x) is concave down, as shown in the graph above. This means thatany instantaneous slope estimated by an interval slope will be anunderestimate(less steep) compared to the instantaneous slope. Sof?(1)>0.4343.

7.Estimatef?(2)forf(x) = 3x. Explain your reasoning.

To estimatef?(2), we selectx= 2 and a point nearby, sayx= 2.0001, and compute the slope over this short interval: f ?(2)?f(2.0001)-f(2)

2.0001-2

3(2.0001)-32

0.0001

?9.888

14.Show how to represent the following on Figure 2.23.

(a)f(4) (b)f(4)-f(2) (c) f(5)-f(2) 5-2 (d)f?(3)

Figure 2.23

2 (a) Height of the function atx= 4. (b) Difference in height betweenf(4) andf(2) (c) Slope/average rate between the points atx= 2 andx= 5. (d) Slope of the tangent line atx= 3

15.For each of the following pairs of numbers, use Figure 2.23 todecide which is larger.

Explain your answer.

(a) or? (b) or? 3 (c)or? (d) or? (a) These both representyvalues, andf(4) is clearly higher thanf(3). (b) These represent differences inyvalues betweenx= 2 and 3, compared tox= 1 and

2. Since the graph is getting flatter further to the right, that means the graph is

growing less quickly, or the samexdistance results in a smallerychange. Thus, f(2)-f(1)> f(3)-f(2) (c) These represent the slopes betweenx= 1 and 2, compared to the slope between x= 1 and 3. By skethching those lines on the graph, the fact thatthe curve is flattening to the right means that the slope over the longer interval will be lower than the slope over the shorter interval. Thus, f(2)-f(1)

2-1>f(3)-f(1)3-1

(d) These are the instantaneous rates of change / slopes of tangent lines atx= 1 and x= 4. Clearly, the graph has a slower rate of change further to the right, so f ?(1)> f?(4) 4