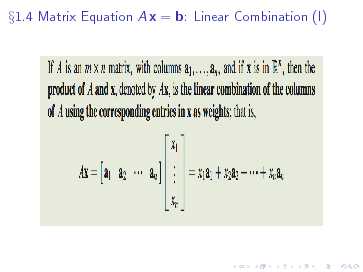
§1.4 Matrix Equation Ax = b: Linear Combination (I)
b2 b3.. . Question: For what values of b1b2
week
18.06 Problem Set 1 Solutions
Feb 11 2010 If E21 subtracts row 1 from row. 2
pset s soln
Math 2331 – Linear Algebra - 1.4 The Matrix Equation Ax=b
1.4 The Matrix Equation Ax = b. Definition Theorem Span Rm. Matrix-Vector Multiplication: Examples. Example 1 −4. 3. 2.
sec
2.5 Inverse Matrices
Elimination solves Ax D b without explicitly using the matrix A. 1 . Note 2 Find the inverses (directly or from the 2 by 2 formula) of A;B;C:.
ila
Now let's show that V ar(aX + b) = a 2V ar(X). This is for a b
Same kind of idea works but just want to remember this. V ar(aX + b) = E((aX + b)2) − (E(aX + b))2. =
prob
Matrix-Vector Products and the Matrix Equation Ax= b
Jan 31 2018 has a solution. 2. Indeed
Lecture
Chapter 2 - Matrices and Linear Algebra
Ax = b. In this way we see that with ci (A) denoting the ith column of A the system is expressible as x1c1 (A) + ··· + xncn (A) = b. From this equation it
chapter
The Matrix Equation Ax = b Section 1.5: Solution Sets of Linear
This section is about solving the “matrix equation” Ax = b where A is an m Exercise 2 (1.7.1): Check if the following vectors are linearly independent:.
ha
Table of Integrals
u(x)v (x)dx = u(x)v(x) v(x)u (x)dx. RATIONAL FUNCTIONS. (5). 1 ax + b dx = 1 a ln(ax + b). (6). 1. (x + a)2 dx = 1 x + a. (7). (x + a)n dx = (x + a)n.
IntegralTable
Math 215 HW #4 Solutions
Execute the six steps following equation (6) to findthee column space and nullspace of A and the solution to Ax = b: A =.. 1 1 2 2. 2 5 7 6. 2 3 5 2.
hw solutions
 x1.4 Matrix EquationAx=b: Linear Combination (I)
x1.4 Matrix EquationAx=b: Linear Combination (I) Matrix-vector Product()Linear Combination (II)
Example:
A=1 21
05 3 ;x=2 443 73
5 Linear Equations in terms of Matrix-vector Product
Example:
Linear Equations in terms of Matrix-vector ProductExample:
Linear Equations in terms of Linear Combinations
Linear Equations in terms of Linear Combinations
Existence of Solutions
Example:LetA=2
41 3 4
4 26 32735 ;andb=2 4b 1 b 2 b 33
5 Question: For what values ofb1;b2;b3is equationAx=bconsistent?2
41 3 4b
1 4 26b 2 327b33
5(`2) + (4)(`1)!(`2)
(`3) + (3)(`1)!(`3)=)241 3 4b
10 14 10b
2+ 4b1
0 7 5b
3+ 3b13
5(`3)(1=2)(`2)!(`3)=)2
41 3 4b
10 14 10b
2+ 4b1
0 0 0b
3+ 3b11=2(b2+ 4b1)3
5Answer: equationAx=bconsistent()b31=2b2+b1= 0:
Existence of Solutions
Example:LetA=2
41 3 4
4 26 32735 ;andb=2 4b 1 b 2 b 33
5 Question: For what values ofb1;b2;b3is equationAx=bconsistent?2
41 3 4b
1 4 26b 2 327b33
5(`2) + (4)(`1)!(`2)
(`3) + (3)(`1)!(`3)=)241 3 4b
10 14 10b
2+ 4b1
0 7 5b
3+ 3b13
5(`3)(1=2)(`2)!(`3)=)2
41 3 4b
10 14 10b
2+ 4b1
0 0 0b
3+ 3b11=2(b2+ 4b1)3
5Answer: equationAx=bconsistent()b31=2b2+b1= 0:
Existence of Solutions
Example:LetA=2
41 3 4
4 26 32735 ;andb=2 4b 1 b 2 b 33
5 Question: For what values ofb1;b2;b3is equationAx=bconsistent?2
41 3 4b
1 4 26b 2 327b33
5(`2) + (4)(`1)!(`2)
(`3) + (3)(`1)!(`3)=)241 3 4b
10 14 10b
2+ 4b1
0 7 5b
3+ 3b13
5(`3)(1=2)(`2)!(`3)=)2
41 3 4b
10 14 10b
2+ 4b1
0 0 0b
3+ 3b11=2(b2+ 4b1)3
5Answer: equationAx=bconsistent()b31=2b2+b1= 0:
Existence of Solutions
Example:LetA=2
41 3 4
4 26 32735 ;andb=2 4b 1 b 2 b 33
5 Question: For what values ofb1;b2;b3is equationAx=bconsistent?2
41 3 4b
1 4 26b 2 327bx1.4 Matrix EquationAx=b: Linear Combination (I)
Matrix-vector Product()Linear Combination (II)
Example:
A=1 21
05 3 ;x=2 443 73
5 Linear Equations in terms of Matrix-vector Product
Example:
Linear Equations in terms of Matrix-vector ProductExample:
Linear Equations in terms of Linear Combinations
Linear Equations in terms of Linear Combinations
Existence of Solutions
Example:LetA=2
41 3 4
4 26 32735 ;andb=2 4b 1 b 2 b 33
5 Question: For what values ofb1;b2;b3is equationAx=bconsistent?2
41 3 4b
1 4 26b 2 327b33
5(`2) + (4)(`1)!(`2)
(`3) + (3)(`1)!(`3)=)241 3 4b
10 14 10b
2+ 4b1
0 7 5b
3+ 3b13
5(`3)(1=2)(`2)!(`3)=)2
41 3 4b
10 14 10b
2+ 4b1
0 0 0b
3+ 3b11=2(b2+ 4b1)3
5Answer: equationAx=bconsistent()b31=2b2+b1= 0:
Existence of Solutions
Example:LetA=2
41 3 4
4 26 32735 ;andb=2 4b 1 b 2 b 33
5 Question: For what values ofb1;b2;b3is equationAx=bconsistent?2
41 3 4b
1 4 26b 2 327b33
5(`2) + (4)(`1)!(`2)
(`3) + (3)(`1)!(`3)=)241 3 4b
10 14 10b
2+ 4b1
0 7 5b
3+ 3b13
5(`3)(1=2)(`2)!(`3)=)2
41 3 4b
10 14 10b
2+ 4b1
0 0 0b
3+ 3b11=2(b2+ 4b1)3
5Answer: equationAx=bconsistent()b31=2b2+b1= 0:
Existence of Solutions
Example:LetA=2
41 3 4
4 26 32735 ;andb=2 4b 1 b 2 b 33
5 Question: For what values ofb1;b2;b3is equationAx=bconsistent?2
41 3 4b
1 4 26b 2 327b33
5(`2) + (4)(`1)!(`2)
(`3) + (3)(`1)!(`3)=)241 3 4b
10 14 10b
2+ 4b1
0 7 5b
3+ 3b13
5(`3)(1=2)(`2)!(`3)=)2
41 3 4b
10 14 10b
2+ 4b1
0 0 0b
3+ 3b11=2(b2+ 4b1)3
5Answer: equationAx=bconsistent()b31=2b2+b1= 0:
Existence of Solutions
Example:LetA=2
41 3 4
4 26 32735 ;andb=2 4b 1 b 2 b 33
5 Question: For what values ofb1;b2;b3is equationAx=bconsistent?2