§1.4 Matrix Equation Ax = b: Linear Combination (I)
b2 b3.. . Question: For what values of b1b2
week
18.06 Problem Set 1 Solutions
Feb 11 2010 If E21 subtracts row 1 from row. 2
pset s soln
Math 2331 – Linear Algebra - 1.4 The Matrix Equation Ax=b
1.4 The Matrix Equation Ax = b. Definition Theorem Span Rm. Matrix-Vector Multiplication: Examples. Example 1 −4. 3. 2.
sec
2.5 Inverse Matrices
Elimination solves Ax D b without explicitly using the matrix A. 1 . Note 2 Find the inverses (directly or from the 2 by 2 formula) of A;B;C:.
ila
Now let's show that V ar(aX + b) = a 2V ar(X). This is for a b
Same kind of idea works but just want to remember this. V ar(aX + b) = E((aX + b)2) − (E(aX + b))2. =
prob
Matrix-Vector Products and the Matrix Equation Ax= b
Jan 31 2018 has a solution. 2. Indeed
Lecture
Chapter 2 - Matrices and Linear Algebra
Ax = b. In this way we see that with ci (A) denoting the ith column of A the system is expressible as x1c1 (A) + ··· + xncn (A) = b. From this equation it
chapter
The Matrix Equation Ax = b Section 1.5: Solution Sets of Linear
This section is about solving the “matrix equation” Ax = b where A is an m Exercise 2 (1.7.1): Check if the following vectors are linearly independent:.
ha
Table of Integrals
u(x)v (x)dx = u(x)v(x) v(x)u (x)dx. RATIONAL FUNCTIONS. (5). 1 ax + b dx = 1 a ln(ax + b). (6). 1. (x + a)2 dx = 1 x + a. (7). (x + a)n dx = (x + a)n.
IntegralTable
Math 215 HW #4 Solutions
Execute the six steps following equation (6) to findthee column space and nullspace of A and the solution to Ax = b: A =.. 1 1 2 2. 2 5 7 6. 2 3 5 2.
hw solutions
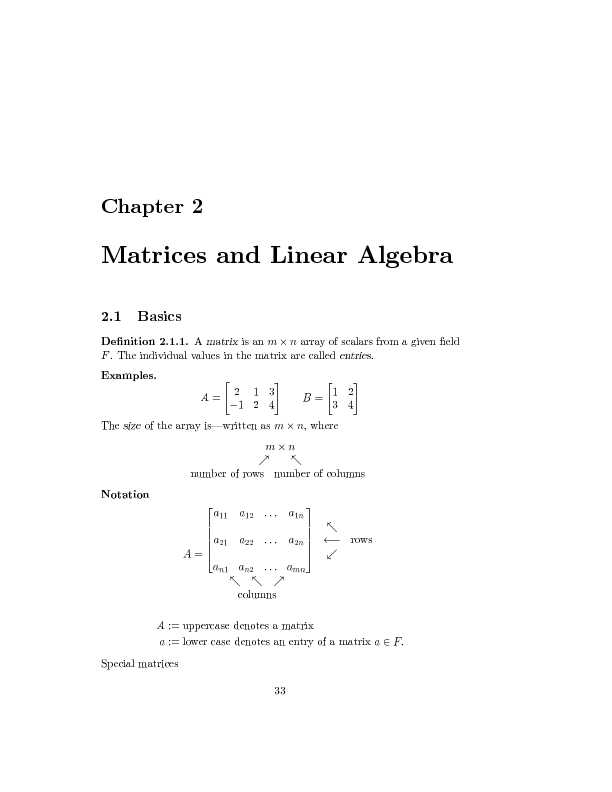
Chapter 2
Matrices and Linear Algebra
2.1 Basics
Definition 2.1.1.Amatrixis anm×narray of scalars from a givenfield F. The individual values in the matrix are calledentries.Examples.
A= 213124
B= 12 34
Thesizeof the array is-written asm×n,where
m×n number of rows number of columnsNotation
A= a 11 a 12 ... a 1n a 21a 22
... a 2n a n1 a n2 ... a mn rows columns
A:= uppercase denotes a matrix
a:= lower case denotes an entry of a matrixaF.Special matrices
3334CHAPTER 2. MATRICES AND LINEAR ALGEBRA
(1) Ifm=n, the matrix is calledsquare.Inthiscasewehave (1a) A matrixAis said to bediagonalif a ij =0i=j. (1b) A diagonal matrixAmay be denoted by diag(d 1 ,d 2 ,...,d n where a ii =d i a ij =0j=i. The diagonal matrix diag(1,1,...,1) is called theidentitymatrix and is usually denoted by I n10...0
01 01 or simplyI,whennis assumed to be known. 0 = diag(0,...,0) is called thezero matrix. (1c) A square matrixLis said to belower triangularif ij =0i0alli,j.
It is calledpositiveif
a ij >0alli,j. Each of these matrices has some special properties, which we will study during this course.2.1. BASICS35
Definition 2.1.2.The set of allm×nmatrices is denoted byM m,n (F), whereFis the underlyingfield (usuallyRorC). In the case wherem=n we writeM n (F) to denote the matrices of sizen×n.Theorem 2.1.1.M
m,n is a vector space with basis given byE ij ,1i m,1jn.Equality, Addition, Multiplication
Definition 2.1.3.Two matricesAandBare equal if and only if they have thesamesizeand a ij =b ij alli,j. Definition 2.1.4.IfAis any matrix andFthen the scalar multipli- cationB=Ais defined by b ij =a ij alli,j. Definition 2.1.5.IfAandBare matrices of the same size then thesumAandBis defined byC=A+B,where
c ij =a ij +b ij alli,j We can also compute thedierenceD=ABby summingAand (1)BD=AB=A+(1)B.
matrix subtraction. Matrix addition "inherits" many properties from thefieldF.Theorem 2.1.2.IfA,B,CM
m,n (F)and,F,then (1)A+B=B+Acommutivity (2)A+(B+C)=(A+B)+Cassociativity (3)(A+B)=A+Bdistributivity of a scalar (4) IfB=0(a matrix of all zeros) thenA+B=A+0=A
(4)(+)A=A+A36CHAPTER 2. MATRICES AND LINEAR ALGEBRA
(5)(A)=A (6)0A=0 (7)0=0.Definition 2.1.6.IfxandyR
n x=(x 1 ...x n y=(y 1 ...y nThen the scalar
or dotproduct ofxandyis given by x,y= nChapter 2
Matrices and Linear Algebra
2.1 Basics
Definition 2.1.1.Amatrixis anm×narray of scalars from a givenfield F. The individual values in the matrix are calledentries.Examples.
A= 213124
B= 12 34
Thesizeof the array is-written asm×n,where
m×n number of rows number of columnsNotation
A= a 11 a 12 ... a 1n a 21a 22
... a 2n a n1 a n2 ... a mn rows columns