FONCTION LOGARITHME NEPERIEN
La fonction logarithme népérien notée ln
LogTS
FONCTION LOGARITHME NEPERIEN (Partie 2)
Démonstration : Nous admettons que la fonction logarithme népérien est dérivable sur 0;+∞⎤⎦⎡⎣ . Posons f (x) = eln x . Alors f '(x) = (ln x)'eln x
LogTESL
LOGARITHME NEPERIEN
.. x ∈ IR+. * y = ln x. ⇔ y ∈ IR e y. = x traduit le fait que les fonctions exponentielle et logarithme népérien sont réciproques l'une ...
ln
Utilisation du logiciel Régressi
multiplication ; / : division ; LN : logarithme népérien ; LOG : logarithme décimal ;. SQRT : racine carrée ; SIN : sinus ; COS : cosinus ; TAN : tangente
notice resume regressi
MATLAB : COMMANDES DE BASE Note : lorsqu'applicable l
exp log
matlab
Exponentielle et logarithme
ln(a). Lien exponentielle et logarithme. La fonction exponentielle (de base e) et la fonction logarithme (népérien) sont des fonctions réciproques : leurs
exponentielle et logarithme
La fonction logarithme népérien
3 déc. 2014 On dit que la fonction ln est la fonction réciproque de la fonction exponentielle. Remarque : Cette fonction existe bien car la fonction ...
Cours fonction logarithme neperien
Finances & Maths : le crédit immobilier
13 avr. 2020 ey =1+ x. — Encore une fois il nous faut trouver y
finances maths le credit immobilier
Fonction logarithme népérien - L'essentiel du cours
Fonction logarithme népérien - L'essentiel du cours a) Existence ln x n'existe que si x > 0. ▷ Exemple : La fonction f définie par f(x)=ln(x − 1) n'est
resume cours logarithme
PRINCIPE D'UTILISATION D'UNE TABLE DE LOGARITHMES
Principe d'utilisation (voir la table de logarithmes décimaux) fonction logarithme népérien (notée ln) qui partage la plupart des.
chapitre ln bis
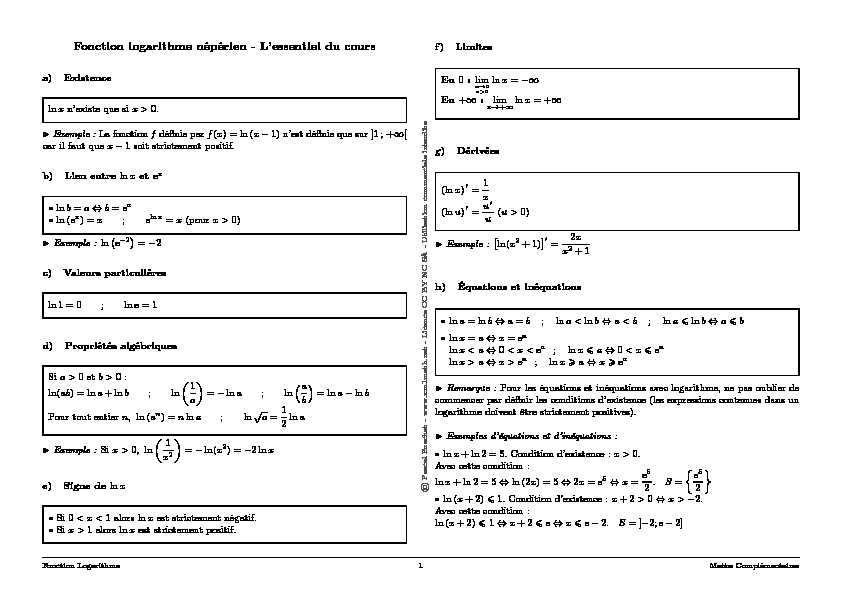
©Pascal Brachet -www.xm1math.net - Licence CC BY NC SA - Utilisation commerciale in terditeFonction logarithme népérien - L"essentiel du cours
car il faut quex-1soit strictement positif. c) Valeurs particulièresln1 = 0;lne= 1d) Propriétés algébriquesSia >0etb >0:ln(ab) = lna+ lnb;ln1a
=-lna;lnab 2 =-ln(x2) =-2lnxe) Signe delnx•Si0< x <1alorslnxest strictement négatif.•Six >1alorslnxest strictement positif.f) Limites
En0:limx→0
x>0lnx=-∞En+∞:limx→+∞lnx= +∞g) Dérivées (lnx)′=1x (lnu)′=u′u 2+ 1 commencer par définir les conditions d"existence (les expressions contenues dans un logarithme doivent être strictement positives). •lnx+ ln2 = 5. Condition d"existence :x >0.Avec cette condition :
lnx+ ln2 = 5⇔ln(2x) = 5⇔2x=e5⇔x=e52 .S=e52 •ln(x+ 2)⩽1. Condition d"existence :x+ 2>0⇔x >-2.Avec cette condition :
ln(x+ 2)⩽1⇔x+ 2⩽e⇔x⩽e-2.S= ]-2;e-2]Fonction Logarithme1Maths Complémentaires
©Pascal Brachet -www.xm1math.net - Licence CC BY NC SA - Utilisation commerciale in terditei) Détermination du plus petit entierntel queqn⩾a(siq >1)ou tel
•Recherche du plus petit entierntel que2n⩾3000: 2 n⩾3000⇔ln(2n)⩾ln(3000)⇔nln2⩾ln3000⇔n⩾ln3000ln2 (carln2>0). Or ln3000ln2 ≈11,55. Le plus petit entier qui convient est donc 12. •Recherche du plus petit entierntel que0,8n⩽0,01:0,8n⩽0,01⇔ln(0,8n)⩽ln(0,01)⇔nln0,8⩽ln0,01⇔n⩾ln0,01ln0,8(car
ln0,8<0). Or ln0,01ln0,8≈20,64. Le plus petit entier qui convient est donc 21. log1 = 0;log10 = 1;log100 = 2 log0,1 =-1;log0,01 =-2Fonction Logarithme2Maths Complémentaires©Pascal Brachet -www.xm1math.net - Licence CC BY NC SA - Utilisation commerciale in terditeFonction logarithme népérien - L"essentiel du cours
car il faut quex-1soit strictement positif. c) Valeurs particulièresln1 = 0;lne= 1d) Propriétés algébriquesSia >0etb >0:ln(ab) = lna+ lnb;ln1a
=-lna;lnab 2 =-ln(x2) =-2lnxe) Signe delnx•Si0< x <1alorslnxest strictement négatif.•Six >1alorslnxest strictement positif.f) Limites
En0:limx→0
x>0lnx=-∞En+∞:limx→+∞lnx= +∞g) Dérivées (lnx)′=1x (lnu)′=u′u 2+ 1 commencer par définir les conditions d"existence (les expressions contenues dans un logarithme doivent être strictement positives). •lnx+ ln2 = 5. Condition d"existence :x >0.Avec cette condition :
lnx+ ln2 = 5⇔ln(2x) = 5⇔2x=e5⇔x=e52 .S=e52 •ln(x+ 2)⩽1. Condition d"existence :x+ 2>0⇔x >-2.Avec cette condition :
ln(x+ 2)⩽1⇔x+ 2⩽e⇔x⩽e-2.S= ]-2;e-2]Fonction Logarithme1Maths Complémentaires
©Pascal Brachet -www.xm1math.net - Licence CC BY NC SA - Utilisation commerciale in terditei) Détermination du plus petit entierntel queqn⩾a(siq >1)ou tel
•Recherche du plus petit entierntel que2n⩾3000: 2 n⩾3000⇔ln(2n)⩾ln(3000)⇔nln2⩾ln3000⇔n⩾ln3000ln2 (carln2>0). Or ln3000ln2 ≈11,55. Le plus petit entier qui convient est donc 12. •Recherche du plus petit entierntel que0,8n⩽0,01: